Hurricanes, flash floods, superstorms. Climate change is bringing more extreme weather, and the results can be catastrophic to our communities. As weather becomes more severe around the globe, there is an increased need for reusable shelters that can be easily transported and assembled to provide relief shelter for families who have been displaced from their homes.

How often is this type of event likely to occur, and who does it affect? In this Science Friday interview, a coastal reporter in New Orleans discusses how millions of people may soon be displaced from coastal regions by storms and sea level rise:

In this activity, you will: 

• Use design thinking to develop a lightweight, flat-folding, structurally-sound shelter for families displaced by natural disasters.
• Incorporate geometric shapes that add strength to structures in your design.
• Practice scaling up your design and converting between metric and U.S. customary systems of measurement.

Share your creations with Science Friday on Twitter or Facebook @SciFri- with the hashtag #DesignAShelter!

Print the student engineering notebook for this activity, or use this digital version. 

Your 2-3 member team has received a plea for help to respond to a natural disaster by developing a prototype foldable shelter.

“Recent severe storms have caused massive flooding across our region.  Thousands of families have been evacuated from their neighborhoods and many homes have been destroyed.  With no way to predict how long these residents will be out of their homes, there is an urgent need for emergency shelters.”

Use this student engineering notebook to lead you through the disaster shelter challenge. The prototype you design for this community must fold flat so that it can be easily transported to the disaster area; yet it must use folds, geometric shapes, and building techniques to make the structure strong enough to provide adequate shelter from wind, rain, and sun.

For practice

• 5 index cards
• 50cm masking tape
• scissors

For prototype

• 2 (used) manilla folders
• 50cm masking tape
• Scissors
• 1kg weight
• Ruler
• Student engineering notebook

Who are the people you are helping?

• What age?
• Are they single people? Families? Are there pets?

What are their needs?

• Will they need multiple rooms?
• Do the users have special needs for their home like a ramp, porch, or multiple doors?

How are they feeling now?

• How would you feel after your home had been destroyed by a natural disaster?
• What sorts of housing amenities would help you and your family feel safe and comforted after losing your home?

What is the problem you are trying to solve? You have been tasked with building a prototype shelter that meets the following criteria:

• Able to fold and pack flat for easy transport.
• Made of 2 manilla folders, using folds to make structure strong enough to hold 1kg without breaking.
• Assembled in under 5 minutes with just tape.
• Be > 2 cm above ground.
• Have ventilation that opens to take advantage of winds, and closes if rains continue.
• Use at least one triangle or column for strength.
• Have 100cm² of floor space to fit a family of 4.

What additional needs did you identify above that you want to focus on in your design? What will define a successful prototype?

Challenge students to come up with their own design constraints based on the needs of the community they defined above. Do they want to have multiple rooms to house a family? Do they want to have windows that close from the inside? Should their structure include a front porch or garage? Additional needs can be described on the student engineering notebook.

How can a simple piece of paper become strong enough to create a shelter? By folding, rolling, or connecting paper we can change its stiffness, and build strong structures out of flexible paper.

Try Out Different Shapes:

Using three index cards and tape, try to fold an index card so that it can hold a book off the table. Experiment with different shapes, heights, and folding patterns, folding each card differently until you find a design that successfully holds a book off the table without crushing the index card.

• Observe your designs and those of your classmates. Which ones were successful at supporting the weight of the book, and why?  

Look around you—what shapes do you see used for strength in buildings, bridges, towers, or products? Most rooms are built in a rectangular shape for aesthetics or convenience, but if you could peer behind the wall, you might see hidden shapes that are supporting the structure.  Geometric shapes such as triangles and cylinders are integral to the support structure of buildings. How do these common shapes add strength to a design?

Generate Ideas Part 1: Build With Triangles

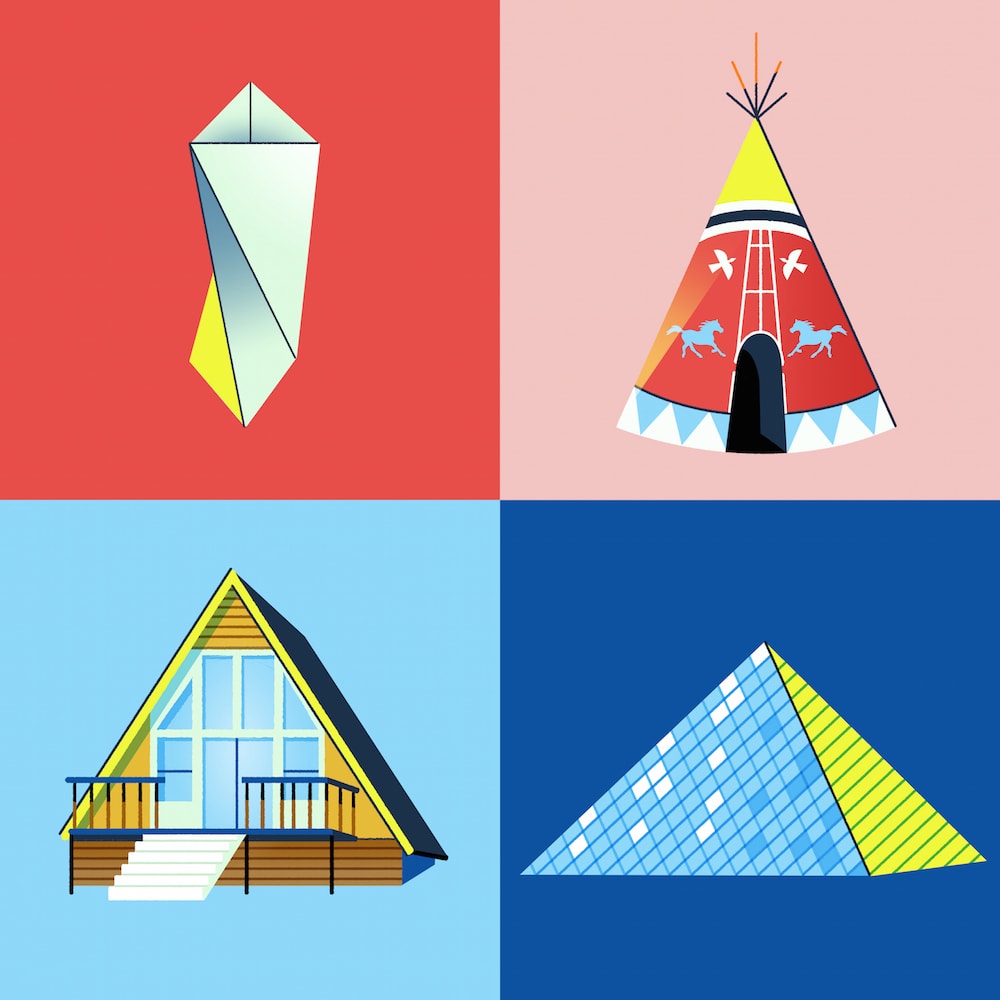
Why are triangles strong? When you push on a square, you can change the angles of the square without bending, or deforming, the sides of the square. Triangles add strength to structures because they resist collapse when pressure is applied to one of their sides.

Triangles can also be used to add strength to weaker shapes. For example, adding a diagonal support to the corner of a rectangle will brace the two sides, adding strength to the structure.

Try folding a new index card into triangles. Test its strength in different directions by loading it with books. What do you notice? In your engineering notebook, write about how you could incorporate triangles into your design.

Generate Ideas Part 2: Building With Cylinders

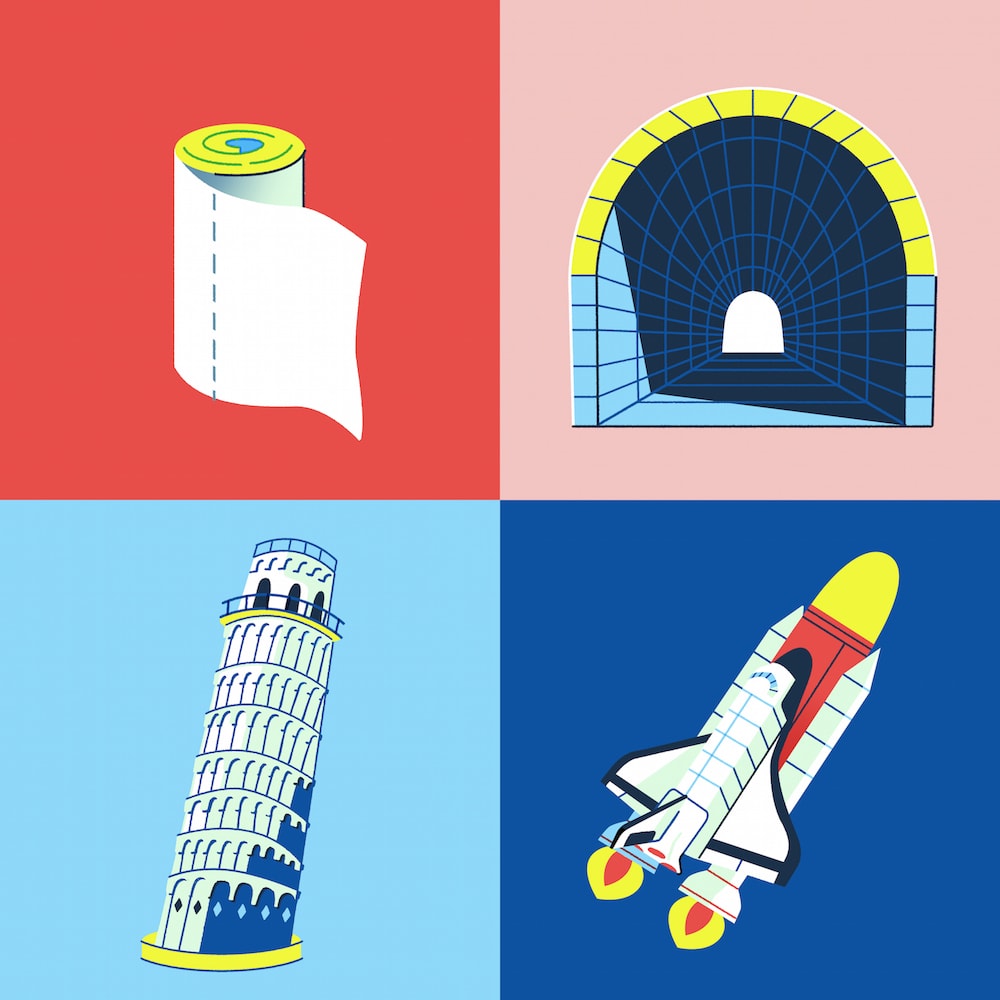
Why are cylinders strong? Cylinders are strong because the forces are dispersed throughout the whole shape. Any shape is only as strong as its weakest point, and since a cylinder distributes the force equally to all points, the shape is much stronger than the same material folded in other ways. In other words, because there is no obvious weak point, cylinders will resist collapse until enough pressure is put on them that they crumple or bow out.If you could fold your paper into a perfect cylinder it would be even stronger.

Try rolling a new index card into a cylinder. Test its strength in different directions by loading it with books. What do you notice? In your engineering notebook, write about how you could incorporate cylinders into your design.

Triangles And Cylinders In Architecture
The use of triangles and cylinders to add strength to structures goes far beyond paper.  Engineers use these same shapes to add strength to to buildings, towers, and bridges. Even materials we think of as very strong (like steel) can bend. Forming these materials into stronger shapes can give them added stiffness and strength. Check out these creative structures that incorporate triangles and cylinders:

Now that you’ve experimented with adding strength to paper through the use of specific shapes, it’s time to apply what you’ve learned to your shelter design. Incorporate what you’ve learned about triangles and cylinders into the design of your prototype shelter. How will you integrate cylinders and triangles into your design?

In your engineering notebook, make a drawing of your final shelter design.  Annotate the drawing of your design to answer the following questions:

1. What will your final structure look like?
2. What are the steps to fold it?
3. How will it pack flat?
4. What design elements will give it strength?

Using your annotated drawing, construct your prototype using the following materials:

• 2 (used) manilla folders
• 50cm masking tape
• Scissors
• 1kg weight
• Ruler

Once you have constructed your prototype, use the engineering notebook to document how well your structure meets the design constraints.

Evaluate whether your prototype has met each of the following design constraints, answering the provided questions on the student engineering notebook.

1. Can be folded and packed flat for easy transport.
   What are the dimensions of your prototype when fully assembled? When packed flat?
2. Uses folds to make two manilla folders into a structure strong enough to hold 1kg without breaking.
   How much mass can your structure hold before collapsing?
   
3. Can be assembled in under 5 minutes using only tape.
   How much time did you need to assemble your prototype?
4. Floor is >2cm off the ground.
   How far off the ground is the floor of your structure?
5. Has ventilation that opens to take advantage of breezes and closes if rains continue.
   Draw your system for opening and closing windows or doors.
6. Has 100cm² of floor space.
   Calculate the square footage of your prototype.
7. Uses at least one triangle or cylinder for strength.
   What shapes did you choose to use for strength and where did you place them?
8. Your group’s design constraints:
   What design constraints did your group choose to add and how did you integrate them into your design?

• Describe the design elements that worked well in your prototype. What are you proud of?
• Discuss the problems with your prototype, and how you might solve them.
• Describe three design elements from other shelters made by other groups that worked well and why.
• As a class, analyze elements from several designs to come up with a new solution that is even better. Work together to create and build a design using the best elements from the structures you tested.

For your prototype to be scaled up to full size, we will need to decide on a conversion that will be large enough to provide actual shelter for a family in need while still fitting on the back of a flatbed truck for transport. Most flatbed trucks in the U.S.A are 11 feet long and 8.5 feet wide. In this section, we will convert from metric to U.S. customary units and then scale up your design to full size.

Conversions
When you evaluated your prototype, you made careful measurements of the dimensions. Your measurements were made in millimeters, but the dimensions of the truck used for transport are in U.S. customary.  Convert your metric measurements in millimeters to inches (for help, try this guide or use this online calculator). Show all work on conversions and enter your results in the engineering extension notebook (find a digital version here). 

Scale Up
Using proportions, determine an appropriate scale for your structure (here’s a guide to scale factors to help you).  Remember, – your scaled up structure must fit onto a flatbed truck when folded flat.  Compute the scaled length, width, and height in the engineering extension notebook.

Share your creations with Science Friday on Twitter or Facebook @SciFri- with the hashtag #DesignAShelter!

Additional Extension Activities

• Collect data on class prototypes (volume of structure, centimeters above ground, weight it can hold) to reflect on characteristics of the various solutions that could be combined into a better structure.
• Combine the best features of group projects to build a larger prototype out of cardboard to fit four real people.
• Incorporate this Science Friday memory wire resource to make a structure that assembles itself

MS-ETS1-1
Define the criteria and constraints of a design problem with sufficient precision to ensure a successful solution, taking into account relevant scientific principles and potential impacts on people and the natural environment that may limit possible solutions.

MS-ETS1-2
Evaluate competing design solutions using a systematic process to determine how well they meet the criteria and constraints of the problem.

Grade 8 Geometry
Solve real-world and mathematical problems involving volume of cylinders, cones, and spheres.

Grade 8 Expressions and Equations
Understand the connections between proportional relationships, lines, and linear equations.

Grade 7 Ratios and Proportional Relationships
Analyze proportional relationships and use them to solve real-world and mathematical problems.

CCSS.Math.Content.7.RP.A.2
Recognize and represent proportional relationships between quantities.

Grade 7 Geometry
Draw construct, and describe geometrical figures and describe the relationships between them.

CCSS.Math.Content.7.G.A.1
Solve problems involving scale drawing of geometric figures, including computing actual lengths and areas from a scale drawing and reproducing a scale drawing at a different scale.

CCSS.Math.Content.7.G.B.6
Solve real-world and mathematical problems involving area, volume and surface area of two- and three-dimensional objects composed of triangles, quadrilaterals, polygons, and right prisms.

CCSS.MATH.CONTENT.7.G.A.1
Solve real-life and mathematical problems involving angle measure, area, surface area, and volume.

Written by: Katie Brown
Editing: Ariel Zych, Johanna Mayer
Digital production: Johanna Mayer, Brian Soash